Math Ref Forum Review by llofte. For more Forum Reviews, see the TiPb iPhone App Store Forum Review Index!
Math Ref is what its name implies - a math reference app. It includes formulas, tips, and examples from many areas of mathematics. Math Ref has the potential to be a very helpful tool once some errors are fixed and the organization becomes more intuitive.
General
Upon launching Math Ref, you are given a list of topics (described in more detail later). Tapping on a topic will give a list of sub-topics. Choosing one of these sub-topics will provide a page of definitions, formulas, figures, and equations relevant to the chosen topic. Tapping a definition, formula, figure, or equation will usually give more information about it. Many times this information is some historical background about the topic, which I think is great. The following screenshots show the sequence of Topics > Algebra > Exponential & Logs > Special Element 1.
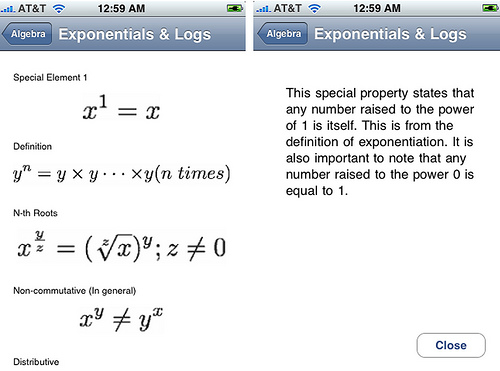
This brings me to my first "complaint". The description should technically say: "It is also important to note that any nonzero number raised to the power 0 is equal to 1." However, I'm willing to agree that I'm just being picky.
Under each topic, there is also an option to view examples. These examples are generally written very well and do a good job of describing the steps that are taken. Just as with formulas, you can tap an example to find out more about it. Here's the screenshots of a derivative example and it's description.
Topics and Organization
Math Ref covers the following areas of mathematics:
- Algebra - addition & multiplication, exponential & logs, formulas, quadratic equation
- Geometry - lines, shapes (2D/3D), surfaces
- Trigonometry - functions, Pythagorean & ratio identity, laws of sines & cosines, double angle identities, half angle identities, sum & difference identities, composite identities, unit circle
- Linear Algebra - definition, addition & multiplication, properties, determinant, 2D transforms, eigenvectors
- Series & Sequences - definitions, properties, sums of powers, geometric, harmonic, alternating, telescoping, power, binomial, binomial coefficients, functions, taylor, Laurent
- Differentiation - definitions, methods, table of derivatives
- Integration - definition, properties, methods, numerical
- Table of Integrals - polynomial, trigonometric, inverse trigonometric, hyperbolic trigonometric, exponential & logarithmic
- Vector Calculus - definitions, dot product, cross product, coordinate conversions, differentials, gradient, divergence, curl
- Differential Equations - linear, separable, homogeneous, inhomogeneous, exact, Bernoulli, real repeated & complex, undermined coefficients, reduction of order
- Discrete - propositions, quantifiers, gates, sets, relations, permutations & combinations, induction
The first thing I noticed when starting to use the app is that there are way too many sub-topics within each topic. For example, the various types of trigonometric identities should be grouped into a single sub-topic. Many times, a student will be using most of the identities during a single homework assignment and going back and forth between sub-topics would get annoying.
The next organization style that I don't like is that lines are grouped under Geometry. I have not heard of a single geometry course that teaches how to find equations of lines. This is taught in algebra classes, and so lines should be in the Algebra topic.
The last thing about the layout I find disappointing is the fact that landscape mode generally doesn't make a difference. It would be nice if landscape mode took advantage of the wider screen and increased the text size. Here's a screenshot to show what I mean.
Errors/Confusion
I found a couple errors in MathRef and consider this terribly inexcusable. Users need to be able to trust that apps like this are actually providing them with accurate information. I'm concerned that there may be other errors that I did not notice.
- Under Discrete > Relations, you can find the definitions to surjective and injective. They are reversed! The definition they provide for surjective is actually the definition for injective. However, when you tap on surjective for more information, you are given the correct definition. The situation is similar for injective. Very confusing!
- Under Linear Algebra > Addition & Multiplication, the definition of matrix multiplication is technically correct, but incredibly vague and incomplete. When tapping for more information, it tells you that the size of the matrices are crucial, but never teaches what size matrices need to be when multiplying them. Matrix multiplication is defined by Math Ref as multiplying the columns of the first matrix with the rows of the second - but how does one multiply rows and columns and where does the answer go in the resulting matrix? All of this needs to be explained.
- Under Differentiation > Table of Derivates, the notation is confusing and simply incorrect. (d/dx) should be used instead of (dy/dx).
- Under Geometry > Shapes (2D/3D) the definitions and figures for the areas of regular polygons do not label what a is. Likely a student will remember that a represents the distance to the center, but there are two different distances with regular polygons - which one do we use?
Under Geometry > Shapes (2D/3D) the definitions and figures for the areas of regular polygons do not label what a is. Likely a student will remember that a represents the distance to the center, but there are two different distances with regular polygons - which one do we use? I have attached screenshots of the errors at the end.
Conclusion
Although my review has been primarily negative, I DO think this is a wonderful app. The things that are correct are done very well and the examples are helpful. I think after a few updates, Math Ref will be awesome. However, because of the errors and unintuitive & inefficient organization, I give Math Ref 3 out of 5 stars. (Fixing the errors alone will bring the score up to 4).
Pros
- Many examples with well written explanations
- Covers many areas of mathematics
- Lots of information
Cons
- Several errors or confusing definitions
- Landscape is generally the same format as portrait
- Some unintuitive and inefficient organization
TiPb Review Rating
Math Ref is available for $0.99 from the iTunes App Store.
